Calculus 1
Collapse
All Calculus 1LimitsDefinition of the DerivativeProduct and Quotient RulePower Rule and Basic DerivativesDerivatives of Trig FunctionsExponential and Logarithmic FunctionsChain RuleInverse and Hyperbolic Trig DerivativesImplicit DifferentiationRelated Rates ProblemsLogarithmic DifferentiationGraphing and Critical PointsOptimization ProblemsIndeterminate Forms and l'Hospital's RuleLinear Approximation and DifferentialsNewton Raphson MethodIndefinite IntegralsU SubstitutionDefinite Integrals and Fundamental TheoremApplications of Integration
AllVideoEasyMediumHardNeeds Solution
A cylindrical tank is full of water and its radius is 3 feet and height is 8 feet. Find the work done pumping the water out of the tank through a pipe which rises 5 feet above the top of the tank. Assume that water weighs 62.5 lbs/ft^3
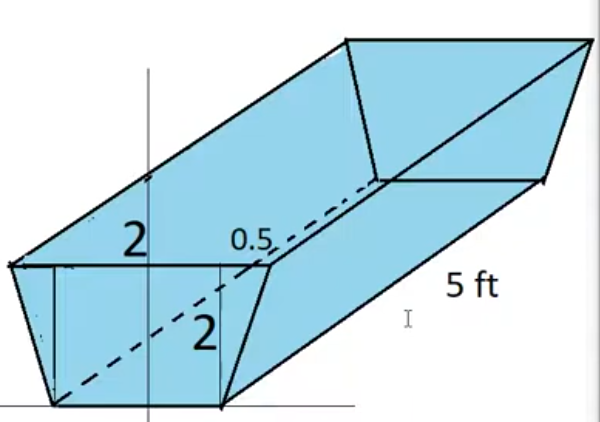
The water trough is 5 feet long and its ends are trapezoids as shown. If the water trough is full of water, find work done in pumping the water out over the top. Assume that water weighs 62.5 lbs/ft^3.
