Calculus 1: Optimization Problems
Collapse
All Calculus 1LimitsDefinition of the DerivativeProduct and Quotient RulePower Rule and Basic DerivativesDerivatives of Trig FunctionsExponential and Logarithmic FunctionsChain RuleInverse and Hyperbolic Trig DerivativesImplicit DifferentiationRelated Rates ProblemsLogarithmic DifferentiationGraphing and Critical PointsOptimization ProblemsIndeterminate Forms and l'Hospital's RuleLinear Approximation and DifferentialsNewton Raphson MethodIndefinite IntegralsU SubstitutionDefinite Integrals and Fundamental TheoremApplications of Integration
AllHardEasyMediumVideoNeeds Solution
An open box is made by cutting and folding a 10x10 inch square of cardboard as shown below. Find the maximum volume the open box can attain by altering the length of cuts, x.

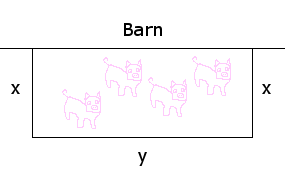
A dudebro has 500 feet of fencing to make a pig pen along a barn as shown below. Find the maximum area.