Product Rule For Exponents

Find the derivative of $2^{3x} \cdot 5^{4x^2}$

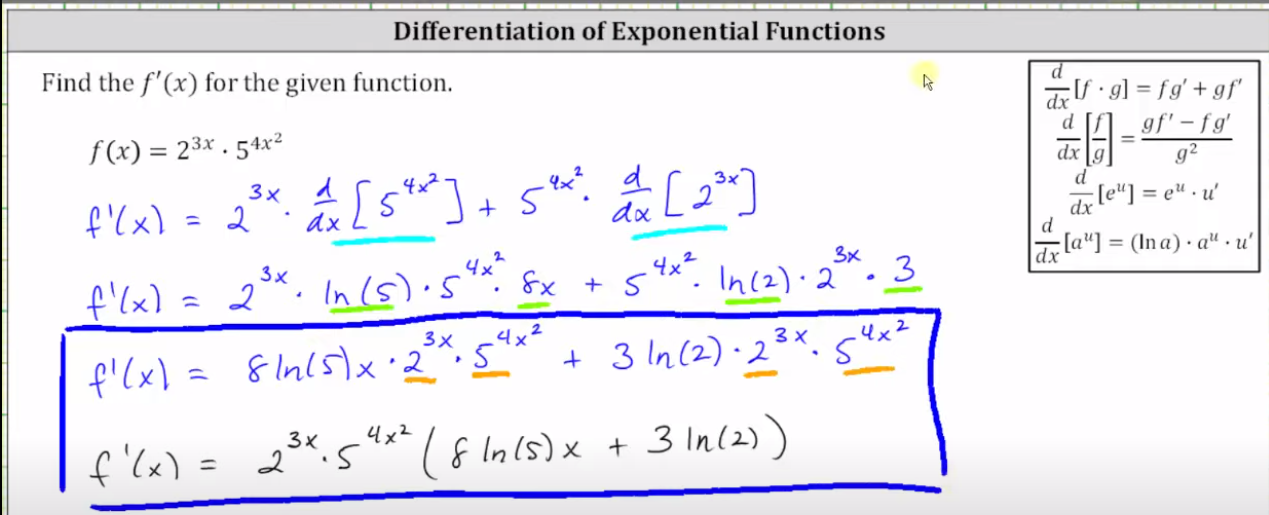
The Product Rule, attributed to Gottfried Leibniz, is a fundamental principle in calculus that facilitates the differentiation of functions being multiplied together. Put simply, it allows us to compute derivatives for expressions that are products of two functions. By leveraging our understanding of both the power rule and the sum and difference rule for derivatives, the product rule states that the derivative of a product of two functions is determined by taking the first function multiplied by the derivative of the second, and adding it to the second function multiplied by the derivative of the first. This rule is invaluable when dealing with functions that cannot be quickly or easily multiplied directly.

$(uv)' = u'v + uv'$

$\left(\frac{u}{v}\right)' = \frac{u'v - uv'}{v^2}$

$(e^u)' = e^u \cdot u'$

$(u^n)' = nu^{n-1}u'$

$(f(g(x)))' = f'(g(x)) \cdot g'(x)$

Related Problems