Moment of Inertia Disk
A solid disk of unknown mass and known radius R is used as a pulley in a lab experiment as shown below. A small block of mass m is attached to a string, the other end of which is attached to the pulley and wrapped around it several times. The block of mass m is released from rest and takes a time t to fall the distance D to the floor.

D. The value of acceleration found in (B)iii, along with numerical values for the given quantities and your answer to (C), can be used to determine the rotational inertia of the pulley. The pulley is removed from its support and its rotational inertia is found to be greater than this value. Give one explanation for this discrepancy.

Rotational inertia, also known as the moment of inertia, is a fundamental concept in rotational dynamics that quantifies an object's resistance to changes in its rotational motion around a specific axis. It depends not only on the mass of the object but also on how that mass is distributed relative to the axis of rotation. Objects with mass further from the axis have higher rotational inertia, making them harder to spin or stop spinning. This principle is analogous to linear inertia in translational motion, where an object's resistance to changes in its state of motion is dependent solely on its mass. Understanding rotational inertia is crucial for solving problems involving rotational kinematics and dynamics, such as calculating angular momentum, torque, and rotational energy.
The rotational inertia (I) of a solid disk is given by the formula: I = 0.5 * M * R^2. Here we don't know the mass of the disk but can find it using the equations of motion and the principle of conservation of energy.
Related Problems
A flywheel turns through 40 rev as it slows from an angular speed of 1.5 rad/s to stop.
A. Assuming constant angular acceleration find the time for it to come to rest.
B. What is its angular acceleration?
C. How much time is required for it to complete its first 20 of the 40 revolutions?
A massless string is wrapped around a disk of rotational inertia I = . The disk falls and spins as it falls, unraveling the rope. Determine the tension in the string and the acceleration of the disk.

A solid brass sphere of mass m and radius r << R rolls along a track when released from rest along the straight section. The circular loop has a radius R.

B. Assume the sphere is released at height h = 6.0 R, what are the magnitude and direction of the horizontal force competent acting on the sphere at point Q?
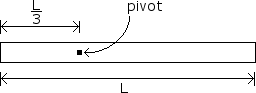
A uniform rod of mass M and length L is attached to a pivot of neglible friction as shown below. The pivot is located at a distance from the left end of the rod. Express all answers in terms of the given quantities and fundamental constants.