Physics 2: Electric Forces and Fields
Two point charges, q = 6Q and q = -2Q, are separated by a distance, d. The attractive force between these two particles is 20 N. If the distanced is halved, so d = d/2, what is the new force between the particles?
Two metal spheres are charged, q = 6Q and q = -2Q, and are separated by a distance d. The attractive force between these two spheres is 20 N. The metal spheres are then brought together so they are touching, and then separated again a distance d. What is the new charge on the metal spheres and what is the new force between them?
Two objects, whose charges are +3 nC and -2 nC, are separated by 1.0 mm. Find the magnitude of the attractive force that either charge exerts on the other.
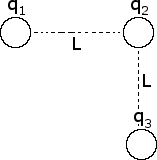
In the figure below, L = 1.0 mm, q = +3 nC, q = -2 nC, and q is +4 nC. Find the magnitude and direction of the net electrostatic force on q
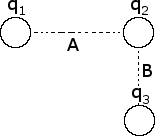
In the figure below, A = 3 cm, B = 1 cm, q = +15 nC, q = -5 nC, and q is -10 nC. Find the magnitude and direction of the net electrostatic force on q
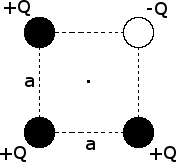
What is the direction of the electric field at the center of the square?
A 100 g ball carries a charge of +0.1 C and is placed in an uniform electric field, E = 10 N/C. Find the acceleration of the ball.
An uncharged metal sphere is place in a uniform electric field as shown below. Which way will the sphere be pushed? Explain.
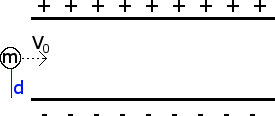
Two charged plates are set up as pictured below. A charged ball of mass m and charge q is to be thrown between the plates with initial velocity V. The ball is a vertical distance, d, from either of the plates. Find how far down range the ball travels.
A charged ball of mass m and charge Q is hung from a mass less pendulum. A uniform electric field along the horizontal causes the pendulum to swing up an angle . Find the magnitude of the electric field.

A proton ( 1.6 x 10 C ) is placed 2.19 x 10 m from point A. Find the electric field at point A. Imagine a proton is put at point A. Find the force that acts on the proton.
A +12 nC charge is distributed uniformly along the y-axis from y = 0 to y = 4.0 m. What is the x-competent of the electric field at x = 2.0 m on the x-axis? (this type of problem may not be covered in algebra based physics courses)
A +1 nC charge is placed at the origin, < 0 , 0 > . Find the electric field at point x = < 0.2 , -0.4 > (this type of problem may not be covered in algebra based physics courses)
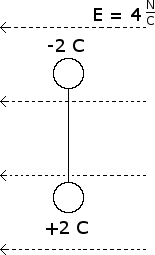
Two point charges are attached by a weightless pole and placed into an electric field, as shown below. Find the torque due to the electric field, E = 4 N/C.
Two parallel charged plates, one with a surface charge density of = 40 and another, = -60 . Find the total electric field between the plates. (note this is not a capacitor)
An electron is released from rest at the surface of a very large plate of charge density -50 . How long will it take for the charge to move 10 cm?
The electron gun in a television tube is used to accelerate electrons from rest to 3.0 x 10 m/s within a distance of 2.0 cm. What electric field is required? Should the electric field be in the same or opposite direction of the electrons motion?
What electric field (magnitude and direction) is needed to levitate a proton?m = 1.67 x 10 kg
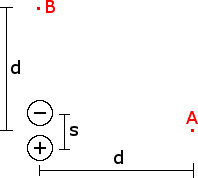
The figure below shows a single dipole of charges 5 nC, with a distance s = 0.002 m between the poles. Point A is a distance, d = 0.1 m, and is perpendicular to the dipole. Point B is a vertical distance, d = 0.1 m, and is parallel to the dipole. Find the electric field at points A and B.
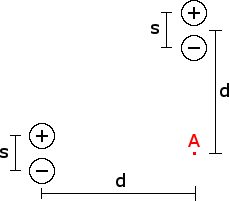
Two dipoles are placed a distance, d = 0.05 m, from the point A (labeled in red). The distance between dipoles is s = 0.01 m. Each dipole consists of a positive and negative charge of 2 nC. Find the electric field at point A.